Why Did a Teacher Mark 5x3=15 Incorrect in America? Reddit Users Were Up in Arms

Illustration - Shutterstock

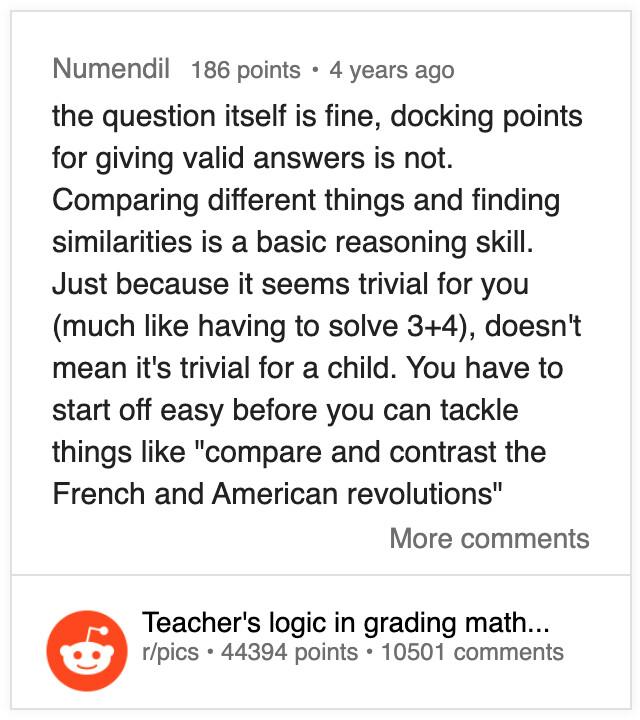
Take a look at these two 3rd-grade math problems, which seem to be answered correctly; but for some reason, the teacher docked marks for them. This question, when posted in 2015 on Reddit, had users up in arms.

When a 3rd-grader correctly answered two questions, “5 x 3 = 15” and “4 x 6 = 24,” and yet both answers still lost marks, Reddit users felt it was either the teacher or the questions that were wrong, not the student, thus arousing an online debate. What do you think? Let’s have a closer look at the problems.

In both questions, the student got the correct solution: 15 and 24, but the questions also asked the student to “use the repeated addition strategy” in question 1, and to “draw an array” in question 2.

While for question 1, the student wrote “5+5+5,” the teacher docked a mark and gave a correction: “3+3+3+3+3.”

For question 2, the student drew six rows of four, and again the teacher docked a mark and gave a correction, drawing four rows of six.

Of course, both add up to the same thing, so why did the student lose two marks just because they arrived at the same answer using only slightly different approaches? When Reddit users saw the corrections, many sided with the student.

Of course, the teacher had a reason for docking the marks; there is a standardized methodology for solving problems in such a way, and clearly the child got the method mixed up, solving it the other way around.

It does raise the question however: Is such standardization necessary in problem solving? Some Reddit users felt it wasn’t, and felt the questions were unfair.

©Reddit

©Reddit

A math teacher who saw the question was in favor of the child’s answer and wrote: “I want to explain why it is detrimental to both the child and the education system to mark it wrong. Firstly, the child has a clear understanding of multiplication as repeated addition. He also shows a clear understanding of commutativity of multiplication.”

The teacher went on to add: “He is also human and doesn’t want to write more than he has to. So what? After having an experience like this the child is less likely to care about his performance and his education in general because of the negative reinforcement of his learning. He displayed multiple ideas correctly and was punished for it.”

READ MORE

• Riddle Time: Second-Grade Math Question Has Everyone Wracking Their Brain
• Relatively Simple Math Problem Goes Viral, Stumps Everyone
• Missing Pieces: Sequencing Math Puzzle Is a Real Head Scratcher, but Can You Solve It?

Meanwhile, another user looked at the problem the way the teacher did and offered an explanation.

The user wrote: “If I asked you for 5 bags that contained three marbles in each bag would you show up with three bags with 5 marbles in each bag and rationalize that it doesn’t matter because there are 15 marbles either way?”

The user also explained that “5x3 using the strategy asked for means 3 is added 5 times. The commutative property is irrelevant because the problem isn’t solely about solving to get the number 15. ”

Illustration - Pixabay | RobinHiggins

The National Council of Teachers of Mathematics (NCTM) in the United States states that, “Part of what we are trying to teach children is to become problem solvers and thinkers,” according to the NCTM president, Diane Briars, as per Daily Mail. “We want students to understand what they’re doing, not just get the right answer.”

Are such bizarre-seeming methodologies important tools for learning or solving problems, or is there a problem with the methodologies that are being taught in classes in America? Are such methodologies useful or are they impediments, indeed real obstacles, in obtaining a true education? What do you think?